AA
Applied Analysis
The Applied Analysis line focuses on physically motivated models, aiming to provide new understanding and insight into the predictions of central physical models, by using rigorous analysis techniques, complemented as needed by numerical simulations.
Current research is concerned with the analytical study of physically motivated systems of partial differential equations. So far it has concentrated on several major directions:
- The Q-tensor theory describing liquid crystals
- The Smoluchowski-Doi models describing complex non-Newtonian fluids
- Aspects of classical Newtonian fluids
- Elliptic systems of phase transition type
- Simulations of solutions of PDEs using neural networks
- Study of fluid models in presence of heterogeneities: variable density, presence of magnetic field, non-homogeneous boundary data...
- Description of the dynamics of geophysical flows
- Study of models from turbulence theory
- Study of low regularity structures
- Analysis of multi-scale processes via singular limits.
The Q-tensor theory and the Smoluchowski-Doi models are relatively new theories, from both a physical and a mathematical point of view. They were proposed in the 70s (the Q-tensor theory), respectively in the 80s (the Smoluchowski-Doi models). They are very popular in the physics community, and in particular Pierre Gilles de Gennes, who proposed the Q-tensor theory got awarded a Nobel Prizes in Physics in 1991 for his work on liquid crystals. Nevertheless they have relatively little mathematical history behind them, most mathematical progress being done in the last decade. On the other hand the nonlinear Schroedinger equation is a firmly established theory with a rich and solid mathematical and physical history.
An equally rich and profound research theme concerns analytical questions related to classical Newtonian fluids. The research so far in this direction has been concerned with wave aspects of ideal flows, respectively computationally relevant analytical questions.
A recent interest is in the Mathematical Design of New Materials theme, which has been the focus of an event co-organised and attended by members of the group at the Isaac Newton Institute, in Cambridge, United Kingdom ( see https://www.newton.ac.uk/event/dnm ).
Another recent interest concerns the applications of machine learning techniques, in particular neural networks to the simulation of solutions of partial differential equations.
Former members of the Applied Analysis team include:
Giacomo Canevari (2017-2019)-currently at University of Verona, Italy.
Arnab Roy (2021-2022)-currently at Technical University of Darmstadt, Germany.
Panayotis Smyrnelis (2019-2022)-currently at University of Athens, Greece.
Stefano Scrobogna (2017-2019)-currently at University of Trieste, Italy.
Jamie Taylor (2018-2022)-currently at CUNEF University, Spain.
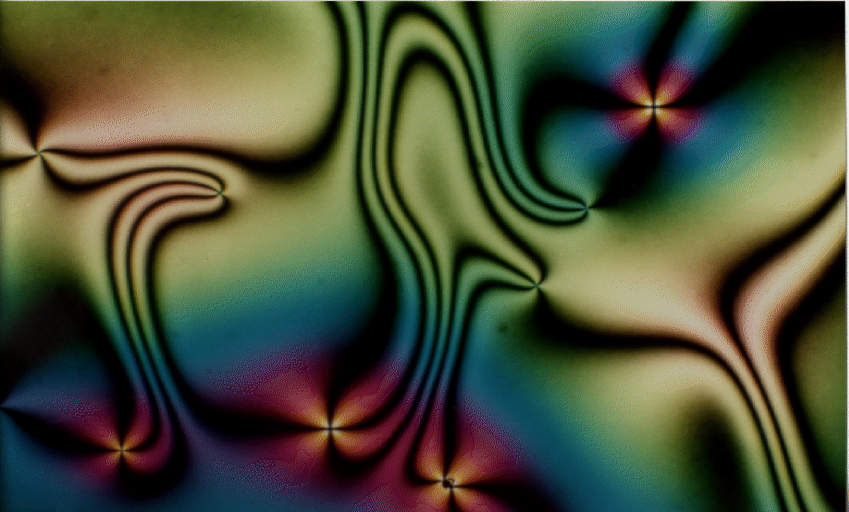
An experiment of Oleg D. Lavrentovich (Kent State University) with defect patterns in nematic liquid crystal sample
