15 urteko ikerketa eta transferentzia

BCAM matematika aplikatuen ikerketa zentroa da, Eusko Jaurlaritzaren eta Euskal Herriko Unibertsitatearen sustapenarekin sortu zena.

Ezagutu BCAM

Job offers

BCAMen, taldeko kide guztien hazkunde profesionala sustatzen dugu. Ezagutu gure azken lan eskaintzak nazioarteko ikerketa proiektuetan parte hartzeko.

Job offers BCAM-en

Events

BCAMek aktiboki parte hartzen du jarduera zientifikoak antolatzen publiko zientifikoarentzat eta, oro har, gizartearentzat. Zentro guztiko ikerketa arloko aktibitateak eta ekintzak aurkitu ahal dituzu. 

Aurkitu hemen hurrengo ekintzak!

Azken berriak

Ikusi guztiak
ESGI

Zentroari buruz

ESGI 188 (European Study Group with Industry) Bilbon izango da 2025eko maiatzaren 26tik 30era

​​​​​​BCAMek Bizkaiko Foru Aldundiarekin eta BAT – B Azeleragailu Dorrearekin elkarlanean antolatutako ekitaldi hau matematikariak eta enpresa industrialak biltzen dituen Europako tailer nagusia da. Edizio honetan lau erronka daude: Lookiero, Quantum Mads, BAT - B Accelerator Tower eta Danobat.…

carlos uriarte

BCAM pertsonak

Carlos Uriarteren tesia, BCAMeko Postdoc Fellow, SEMAk (Sociedad Española de Matemática Aplicada) ECCOMAS sarietarako hautatu du, zientzia aplikatuetako eta ingeniaritzako metodo informatikoei buruzko 2024ko bi doktoretza-tesi onenetarako

  • Carlos Uriarte BCAMeko (Diseinu Matematikoa, Modelizazioa eta Simulazioak) Postdoc bekadunak "Ekuazio Diferentzial Partzialen Ebazpena Neurona-sare Artifizialen bidez" izeneko tesia de

bcam naukas

Zentroari buruz

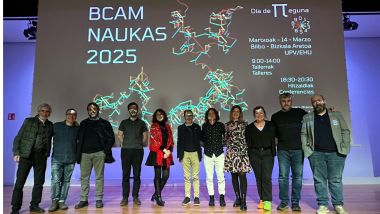
2000 pertsona baino gehiago izan dira BCAM Naukas 2025 jardunaldian Matematikaren eguna ospatzen

  • Seigarren edizioak jarduera-programa zabala izan du, eta matematikak eta haren eguneroko aplikazioek hartu dute prota

ELNAZ

BCAM pertsonak

Elnazossadat Zohravi, BCAMeko doktoretza ondoko bekaduna, Bilboko Udalak aintzatetsia hiriko bizitza sozial eta publikoari egindako ekarpenagatik "Emakume migratu bilbotarren errekonozimendua" ren VI. edizioan

  • Udal ekimen hau Immigrazioko Tokiko Kontseiluaren (CLI) proiektu estrategiko bat da, Bilboko Kultura Arteko Hiri Planaren barruan dagoen bizitza sozial eta publikoan emakume etorkinen

failed to load external entity "https://bird.bcamath.org/feed/rss_2.0/site" failed to load external entity "https://bird.bcamath.org/feed/atom_1.0/site"
failed to load external entity "https://bird.bcamath.org/feed/rss_2.0/site" failed to load external entity "https://bird.bcamath.org/feed/atom_1.0/site" failed to load external entity "https://bird.bcamath.org/feed/rss_2.0/site" failed to load external entity "https://bird.bcamath.org/feed/atom_1.0/site"

There were errors showing the content (1).